[
ATTACH=CONFIG]46705[/ATTACH]
Tomorrow, we have a Winter Fair at church and I am giving some of these to sell. I found the idea on Pinterest and I thought it a good way to use up offcuts, particularly arttractive woods. They were posted as “tsumi-ishi” and can be bought on Etsy from Japan for a surprising amount of dollars.
I was thinking of what to say to the vendor. Customers are likely to want to experiment with stacking them, that’s the idea. But they will have to be tactfully discouraged. Then I realised I had unfortunately forgotten how to calculate the theoretical number of permutations. It is certain that someone on HMT knows.
Assuming that each block has seven faces and a customer is going to emulate one of the columns of 5.
7! ,then, if the order is predetermined. Is it 7! To the power of 5, if any order is allowed?
The stack in the foreground is included, with the base wrapped in masking tape, since the best way of gluing, I found, was “Mitre Mate” , a 5-second setting superglue. But the catalyst has a tendency to wick beneath the paper, marring the appearance.



 LinkBack URL
LinkBack URL About LinkBacks
About LinkBacks


 Reply With Quote
Reply With Quote

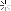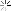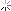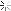

Bookmarks